72 is not a perfect square. It is represented as √72. The square root of 72 can only be simplified. In this mini-lesson we will learn to find square root of 72 by long division method along with solved examples. Let us see what the square root of 72 is.
- Square Root of 72: √72 = 8.4852
- Square of 72: 722 = 5184
1. What Is the Square Root of 72? 2. Is Square Root of 72 Rational or Irrational? 3. How to Find the Square Root of 72? 4. FAQs on Square Root of 72
You are viewing: Which Expression Is Equal To 72
The original number whose square is 72 is the square root of 72. Can you find what is that number? It can be seen that there are no integers whose square gives 72.
√72 = 8.4852
Read more : Which Of The Following Can Be Causes Of Frictional Unemployment
To check this answer, we can find (8.4852)2 and we can see that we get a number 71.99861904. This number is very close to 72 when its rounded to its nearest value.
Any number which is either terminating or non-terminating and has a repeating pattern in its decimal part is a rational number. We saw that √72 = 8.48528137423857. This decimal number is non-terminating and the decimal part has no repeating pattern. So it is NOT a rational number. Hence, √72 is an irrational number.
Important Notes:
- 72 lies between 64 and 81. Hence, √72 lies between √64 and √81, i.e., √72 lies between 8 and 9.
- Square root of a non-perfect square number in the simplest radical form can be found using prime factorization method. For example: 72 = 2 × 2 × 2 × 3 × 3. So, √72 = √(2 × 2 × 2 × 3 × 3) = 6√2.
There are different methods to find the square root of any number. We can find the square root of 72 using long division method. Click here to know more about it.
Simplified Radical Form of Square Root of 72
72 is a composite number. Hence factors of 72 are 1, 2, 3, 4, 6, 8, 9 12, 18, 24, 36, and 72. When we find the square root of any number, we take one number from each pair of the same numbers from its prime factorization and we multiply them. The factorization of 72 is 2 × 2 × 2 × 3 × 3 which has 1 pair of the same number. Thus, the simplest radical form of √72 is 6√2.
Square Root of 72 by Long Division Method
Read more : Which Best Describes The Alternation Of Generations
The square root of 72 can be found using the long division as follows.
- Step 1: In this step, we pair off digits of a given number starting with a digit at one’s place. We put a horizontal bar to indicate pairing.
- Step 2: Now we need to find a number which on squaring gives value less than or equal to 72. As we know, 8 × 8 = 64 < 72. The divisor obtained is 8 and the quotient is 8.
- Step 3: Now, we have to bring down 00 and multiply the quotient by 2 which gives us 16.
- Step 4: 4 is written at one’s place of new divisor because when 164 is multiplied by 4, 656 is obtained which is less than 800. The obtained answer now is 144 and we bring down 00.
- Step 5: The quotient is now 84 and it is multiplied by 2. This gives 168, which then would become the starting digit of the new divisor.
- Step 6: 7 is written at one’s place of new divisor because when 1688 is multiplied by 8, 13504 is obtained which is less than 14400. The obtained answer now is 896 and we bring down 00.
- Step 7: The quotient is now 848 and it is multiplied by 2. This gives 1696, which then would become the starting digit of the new divisor.
- Step 8: 5 is written at one’s place of new divisor because when 16965 is multiplied by 8, 84825 is obtained which is less than 89600. The obtained answer now is 4775 and we bring down 00.
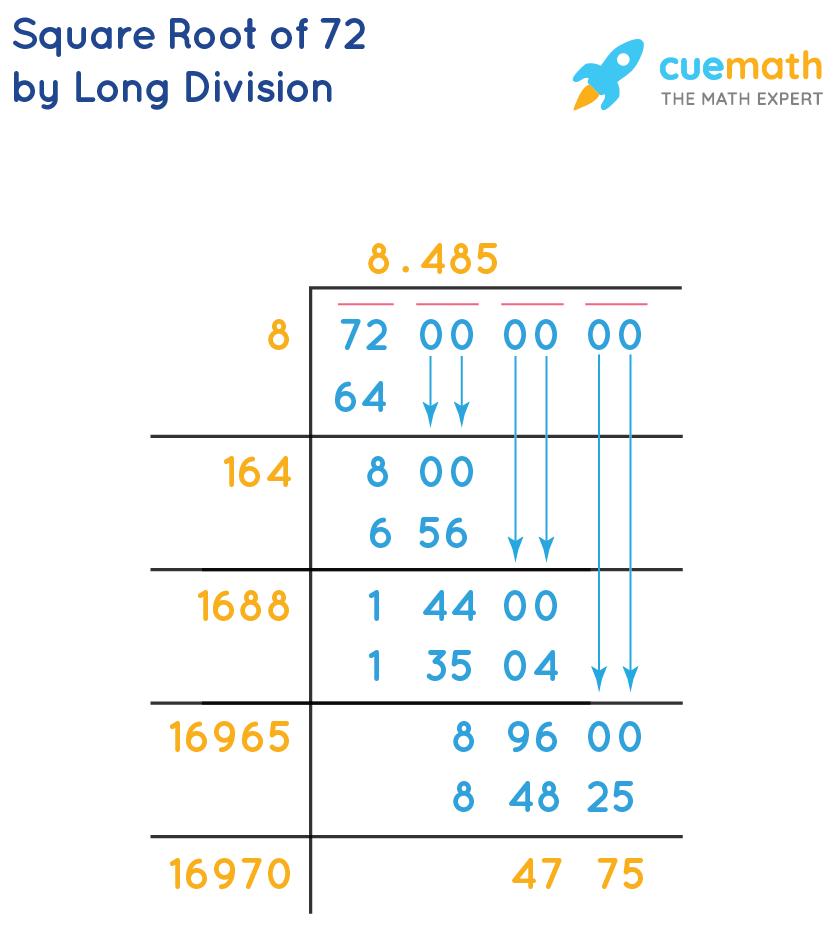
So far we have got √72 = 8.485. On repeating this process further, we get, √72 = 8.48528137423857
Explore square roots using illustrations and interactive examples.
- Square Root of 61
- Square Root of 63
- Square Root of 65
- Square Root of 68
- Square Root of 85
Think Tank:
- Are √-72 and –√72 same ?
- Is √-72 a real number?
Source: https://t-tees.com
Category: WHICH
