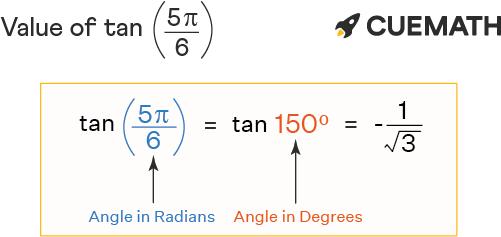
The value of tan 5pi/6 is -0.5773502. . .. Tan 5pi/6 radians in degrees is written as tan ((5π/6) × 180°/π), i.e., tan (150°). In this article, we will discuss the methods to find the value of tan 5pi/6 with examples.
- Tan 5pi/6: -1/√3
- Tan 5pi/6 in decimal: -0.5773502. . .
- Tan (-5pi/6): 0.5773502. . . or 1/√3
- Tan 5pi/6 in degrees: tan (150°)
What is the Value of Tan 5pi/6?
The value of tan 5pi/6 in decimal is -0.577350269. . .. Tan 5pi/6 can also be expressed using the equivalent of the given angle (5pi/6) in degrees (150°).
You are viewing: Which Of The Following Is Equivalent To Tan 5pi 6
We know, using radian to degree conversion, θ in degrees = θ in radians × (180°/pi) ⇒ 5pi/6 radians = 5pi/6 × (180°/pi) = 150° or 150 degrees ∴ tan 5pi/6 = tan 5π/6 = tan(150°) = -1/√3 or -0.5773502. . .
Read more : Which Bank Can I Open Without Bvn
Explanation:
For tan 5pi/6, the angle 5pi/6 lies between pi/2 and pi (Second Quadrant). Since tangent function is negative in the second quadrant, thus tan 5pi/6 value = -1/√3 or -0.5773502. . . Since the tangent function is a periodic function, we can represent tan 5pi/6 as, tan 5pi/6 = tan(5pi/6 + n × pi), n ∈ Z. ⇒ tan 5pi/6 = tan 11pi/6 = tan 17pi/6 , and so on. Note: Since, tangent is an odd function, the value of tan(-5pi/6) = -tan(5pi/6).
Methods to Find Value of Tan 5pi/6
The tangent function is negative in the 2nd quadrant. The value of tan 5pi/6 is given as -0.57735. . .. We can find the value of tan 5pi/6 by:
- Using Unit Circle
- Using Trigonometric Functions
Tan 5pi/6 Using Unit Circle
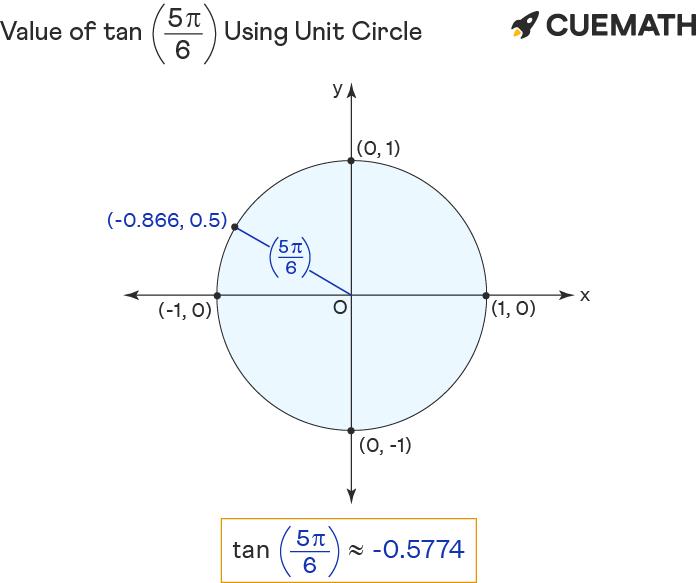
To find the value of tan 5π/6 using the unit circle:
- Rotate ‘r’ anticlockwise to form 5pi/6 angle with the positive x-axis.
- The tan of 5pi/6 equals the y-coordinate(0.5) divided by the x-coordinate(-0.866) of the point of intersection (-0.866, 0.5) of unit circle and r.
Read more : Which Witch Is Which Game
Hence the value of tan 5pi/6 = y/x = -0.5774 (approx)
Tan 5pi/6 in Terms of Trigonometric Functions
Using trigonometry formulas, we can represent the tan 5pi/6 as:
- sin(5pi/6)/cos(5pi/6)
- ± sin(5pi/6)/√(1 – sin²(5pi/6))
- ± √(1 – cos²(5pi/6))/cos(5pi/6)
- ± 1/√(cosec²(5pi/6) – 1)
- ± √(sec²(5pi/6) – 1)
- 1/cot(5pi/6)
Note: Since 5pi/6 lies in the 2nd Quadrant, the final value of tan 5pi/6 will be negative.
We can use trigonometric identities to represent tan 5pi/6 as,
- cot(pi/2 – 5pi/6) = cot(-pi/3)
- -cot(pi/2 + 5pi/6) = -cot 4pi/3
- -tan (pi – 5pi/6) = -tan pi/6
☛ Also Check:
- cot pi
- sec pi/3
- csc pi/6
- cos 7pi/12
- cot 5pi/4
- tan 3pi/2
Source: https://t-tees.com
Category: WHICH
