Which Of The Following Rational Functions Is Graphed Below 5

A rational function is a ratio of polynomials where the polynomial in the denominator shouldn’t be equal to zero. Isn’t it resembling the definition of a rational number (which is of the form p/q, where q ≠ 0)? Did you know Rational functions find application in different fields in our day-to-day life? Not only do they describe the relationship between speed, distance, and time, but also are widely used in the medical and engineering industry.

Let us learn more about rational functions along with how to graph it, its domain, range, asymptotes, etc along with solved examples.

You are viewing: Which Of The Following Rational Functions Is Graphed Below 5

1. What is a Rational Function? 2. How to Identify a Rational Function? 3. Domain and Range of Rational Function 4. Asymptotes of Rational Function 5. Graphing Rational Functions 6. Inverse of a Rational Function 7. FAQs on Rational Functions

A rational function is a function that is the ratio of polynomials. Any function of one variable, x, is called a rational function if, it can be represented as f(x) = p(x)/q(x), where p(x) and q(x) are polynomials such that q(x) ≠ 0. For example, f(x) = (x2 + x – 2) / (2×2 – 2x – 3) is a rational function and here, 2×2 – 2x – 3 ≠ 0.

We know that every constant is a polynomial and hence the numerators of a rational function can be constants also. For example, f(x) = 1/(3x+1) can be a rational function. But note that the denominators of rational functions cannot be constants. For example, f(x) = (2x + 3) / 4 is NOT a rational function, rather, it is a linear function.

By the definition of the rational function (from the previous section), if either the numerator or denominator is not a polynomial, then the fraction formed does NOT represent a rational function. For example, f(x) = (4 + √x)/(2-x), g(x) = (3 + (1/x)) / (2 – x), etc are NOT rational functions as numerators in these examples are NOT polynomials.

Any fraction is not defined when its denominator is equal to 0. This is the key point that is used in finding the domain and range of a rational function.

Domain of Rational Function

The domain of a rational function is the set of all x-values that the function can take. To find the domain of a rational function y = f(x):

• Set the denominator ≠ 0 and solve it for x.
• Set of all real numbers other than the values of x mentioned in the last step is the domain.

Example: Find the domain of f(x) = (2x + 1) / (3x – 2).

Solution:

We set the denominator not equal to zero.

3x – 2 ≠ 0 x ≠ 2/3

Thus, the domain = {x ∈ R | x ≠ 2/3}

Range of Rational Function

The range of a rational function is the set of all outputs (y-values) that it produces. To find the range of a rational function y= f(x):

• If we have f(x) in the equation, replace it with y.
• Solve the equation for x.
• Set the denominator of the resultant equation ≠ 0 and solve it for y.
• Set of all real numbers other than the values of y mentioned in the last step is the range.

Example: Find the range of f(x) = (2x + 1) / (3x – 2).

Solution:

Let us replace f(x) with y. Then y = (2x + 1) / (3x – 2). Now, we will solve this for x.

(3x – 2) y = (2x + 1) 3xy – 2y = 2x + 1 3xy – 2x = 2y + 1 x (3y – 2) = (2y + 1) x = (2y + 1) / (3y – 2)

Now (3y – 2) ≠ 0 y ≠ 2/3

So the range = {y ∈ R | y ≠ 2/3}

A rational function can have three types of asymptotes: horizontal, vertical, and slant asymptotes. Apart from these, it can have holes as well. Let us see how to find each of them.

Holes of a Rational Function

The holes of a rational function are points that seem that they are present on the graph of the rational function but they are actually not present. They can be obtained by setting the linear factors that are common factors of both numerator and denominator of the function equal to zero and solving for x. We can find the corresponding y-coordinates of the points by substituting the x-values in the simplified function. Every rational function does NOT need to have holes. Holes exist only when numerator and denominator have linear common factors.

Example: Find the holes of the function f(x) = (x2 + 5x + 6) / (x2 + x – 2).

Solution:

Let us factorize the numerator and denominator and see whether there are any common factors.

f(x) = [ (x + 2)(x + 3) ] / [ (x + 2) (x – 1) ] = [ ̶(̶x̶ ̶+̶ ̶2̶)̶(x + 3) ] / [ ̶(̶x̶ ̶+̶ ̶2̶)̶ (x – 1) ] = (x + 3) / (x – 1)

Since (x + 2) was striked off, there is a hole at x = -2. Its y-coordinate is f(-2) = (-2 + 3) / (-2 – 1) = -1/3.

Thus, there is a hole at (-2, -1/3).

Vertical Asymptote of a Rational Function

A vertical asymptote (VA) of a function is an imaginary vertical line to which its graph appears to be very close but never touch. It is of the form x = some number. Here, “some number” is closely connected to the excluded values from the domain. But note that there cannot be a vertical asymptote at x = some number if there is a hole at the same number. A rational function may have one or more vertical asymptotes. So to find the vertical asymptotes of a rational function:

• Simplify the function first to cancel all common factors (if any).
• Set the denominator = 0 and solve for (x) (or equivalently just get the excluded values from the domain by avoiding the holes).

Example: Find the vertical asymptotes of the function f(x) = (x2 + 5x + 6) / (x2 + x – 2).

Solution:

We have already seen that this function simplifies to f(x) = (x + 3) / (x – 1).

Setting the denominator to 0, we get

x – 1 = 0 x = 1

Thus, there is a VA of the given rational function is, x = 1.

Horizontal Asymptote of a Rational Function

A horizontal asymptote (HA) of a function is an imaginary horizontal line to which its graph appears to be very close but never touch. It is of the form y = some number. Here, “some number” is closely connected to the excluded values from the range. A rational function can have at most one horizontal asymptote. Easy way to find the horizontal asymptote of a rational function is using the degrees of the numerator (N) and denominators (D).

• If N < D, then there is a HA at y = 0.
• If N > D, then there is no HA.
• If N = D, then the HA is y = ratio of the leading coefficients.

Example: Find the horizontal asymptote (if any) of the function f(x) = (x2 + 5x + 6) / (x2 + x – 2).

Solution:

Here the degree of the numerator is, N = 2, and the degree of the denominator is, D = 2.

Since N = D, the HA is y = (leading coefficient of numerator) / (leading coefficient of denominator) = 1/1 = 1.

Thus, the HA is y = 1.

Slant (Oblique) Asymptotes of a Rational Function

A slant asymptote is also an imaginary oblique line to which a part of the graph appears to touch. A rational function has a slant asymptote only when the degree of the numerator (N) is exactly one greater than the degree of the denominator (D). Its equation is y = quotient that is obtained by dividing the numerator by denominator using the long division.

Example: Find the slant asymptote of the function f(x) = x2/(x+1).

Solution:

Here the degree of numerator is 2 and that of denominator = 1. So it has a slant asymptote.

Let us divide x2 by (x + 1) by long division (or we can use synthetic division as well).

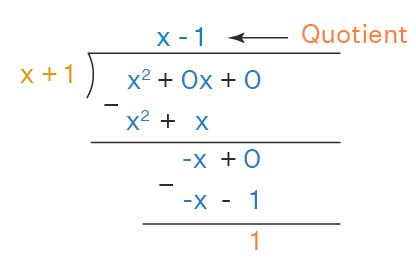
Read more : Which Fire Cable Rating Has No Significant Fire Protection

Thus, the slant asymptote is y = x – 1.

Here are the steps for graphing a rational function:

1. Identify and draw the vertical asymptote using a dotted line.
2. Identify and draw the horizontal asymptote using a dotted line.
3. Plot the holes (if any)
4. Find x-intercept (by using y = 0) and y-intercept (by x = 0) and plot them.
5. Draw a table of two columns x and y and place the x-intercepts and vertical asymptotes in the table. Then take some random numbers in the x-column on either side of each of the x-intercepts and vertical asymptotes.
6. Compute the corresponding y-values by substituting each of them in the function.
7. Plot all points from the table and join them curves without touching the asymptotes.

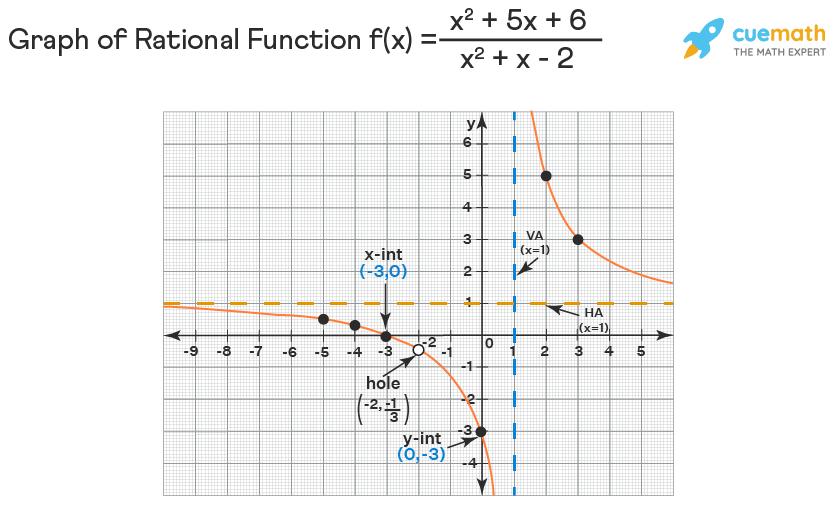
Example: Graph the rational function f(x) = (x2 + 5x + 6) / (x2 + x – 2).

Solution:

We have already identified that its VA is x = 1, its HA is y = 1, and the hole is at (-2, -1/3). We use dotted lines for asymptotes so that we can take care that the graph doesn’t touch those lines. Note that, the simplified form of the given function is, f(x) = (x + 3) / (x – 1). Now, we will find the intercepts.

• For x-intercept, put y = 0. Then we get 0 = (x + 3) / (x – 1) ⇒ x + 3 = 0 ⇒ x = -3. So the x-intercept is at (-3, 0).
• For y-intercept, put x = 0. Then we get y = (0 + 3) / (0 – 1) ⇒ y = -3. So the y-intercept is at (0, -3).

We have the VA at x = 1 and x-intercept is at x = -3. Let us construct a table now with these two values in the column of x and some random numbers on either side of each of these numbers -3 and 1.

x y -5 y = (-5 + 3) / (-5 – 1) = 0.33 -4 y = (-4 + 3) / (-4 – 1) = 0.2 -3 0 (x-int) -2 y = (-2 + 3) / (-2 – 1) = -0.33 0 -3 (y-int) 1 VA 2 y = (2 + 3) / (2 – 1) = 5 3 y = (3 + 3) / (3 – 1) = 3

Let us plot all these points on the graph along with all asymptotes, hole, and intercepts.

To find the inverse of a rational function y = f(x):

• Replace f(x) with y.
• Interchange x and y.
• Solve the resultant equation for y.
• The result would give the inverse f-1(x).

Example: Find the inverse of the rational function f(x) = (2x – 1) / (x + 3).

Solution:

The given function can be written as:

y = (2x – 1) / (x + 3)

Interchanging x and y:

x = (2y – 1) / (y + 3)

Now, we will solve for y.

x(y + 3) = 2y – 1

xy + 3x = 2y – 1

3x + 1 = 2y – xy

3x + 1 = y (2 – x)

y = (3x + 1) / (2 – x) = f-1(x)

Important Notes on Rational Function:

• A rational function equation is of the form f(x) = P(x) / Q(x), where Q(x) ≠ 0.
• Every rational function has at least one vertical asymptote.
• Every rational function has at most one horizontal asymptote.
• Every rational function has at most one slant asymptote.
• The excluded values of the domain of a rational function help to identify the VAs.
• The excluded values of the range of a rational function help to identify the HAs.
• The linear factors that get canceled when a rational function is simplified would give us the holes.

☛ Related Topics:

• Graphing Functions
• Simplifying Rational Expressions Calculator
• Asymptote Calculator
• Reciprocal Function

Source: https://t-tees.com
Category: WHICH