Do you know that the sum of the first five prime numbers, i.e., 2, 3, 5, 7, and 11, is 28? Another interesting fact about 28 is that it is a perfect number because the sum of all the numbers that divide 28 perfectly is 28. 1+2+4+7+14 = 28. Isn’t that interesting? The number 28 is also a triangular number. It means the number of dots in seven rows of triangular dots is 28. In this chapter, we will calculate the square root of 28 by long division method along with solved examples and interactive questions. Let us see what the square root of 28 is.
- Square Root of 28: √28 = 5.29150
- Square of 28: 282 = 784
1. What Is the Square Root of 28? 2. Is Square Root of 28 Rational or Irrational? 3. How to Find the Square Root of 28? 4. FAQs on Square Root of 28
You are viewing: Which Statement Is True About The Square Root Of 28
Square root of 28 in the radical form, is represented as √28, while in the exponent form, it is expressed as (28)1/2. Non-square numbers also have a square root, just that they are not whole numbers. The square root of 28, rounded to 6 decimal places is 5.291502.
A rational number is a number that is of the form p/q where p and q are integers and q is not equal to 0. A number that cannot be expressed as a ratio of two integers is an irrational number. Non-terminating decimals having repeated numbers after the decimal point are rational numbers. √28 = 5.291502322…
Read more : Which Shrek Character Am I
Do you think the decimal part stops after 5.291502322? No, it is never-ending. Therefore, it is a non-terminating decimal with non-repeating numbers. The number 5.291502322… can’t be written in p/q form. So √28 is an irrational number.
Square roots can be calculated using two methods:
- By simplifying the radical of the numbers that are perfect squares
- By using the long division method for perfect and non-perfect squares
The number 28 is lies between 25 and 36, therefore 28 is not a perfect square of an integer. Hence, the long division method is used to evaluate the square root of 28.
Simplified Radical Form of Square Root of 27
To simplify the square root of 28, let us first express 28 as a product of its prime factors. Prime factorization of 28 is 2 × 2 × 7. Therefore, √28 can be simplified further as √(2 × 2 × 7) =2√7. Thus, we have expressed the square root of 28 in the simplest radical form as 2√7.
Square Root of 27 by Long Division Method
Read more : Which Generation Am I Quiz
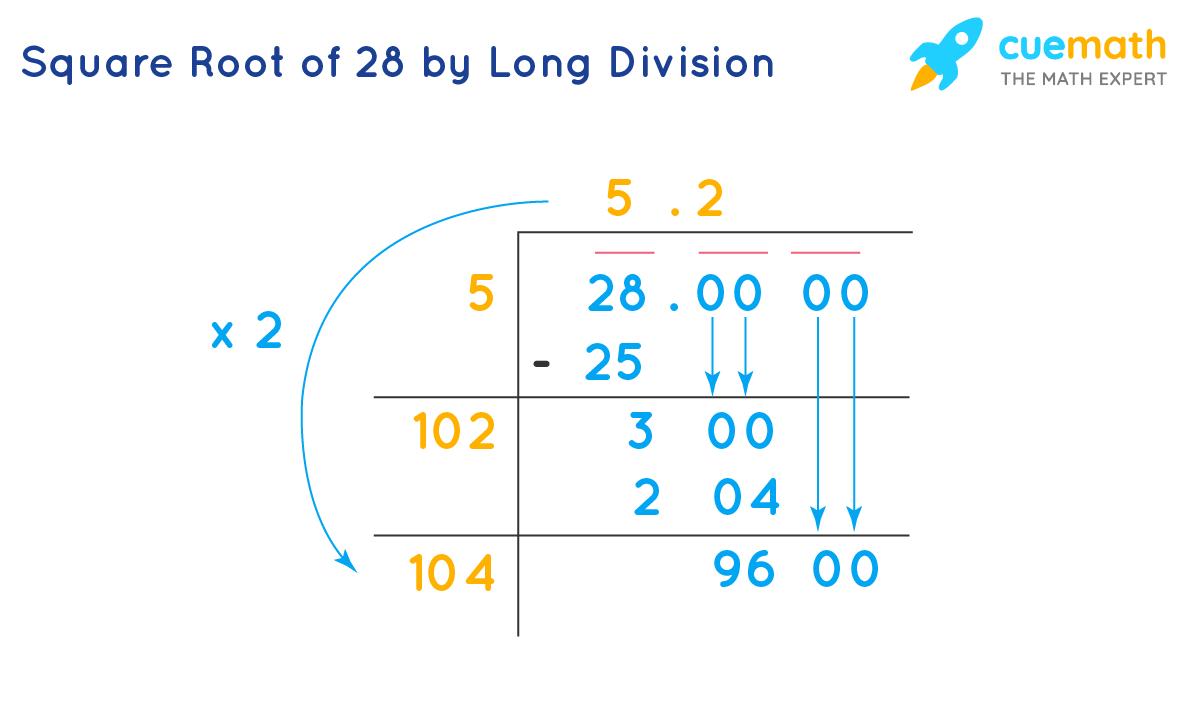
Follow the steps given below to find the square root of 28 by long division.
- Step 1: We group the digits 2 and 8 into a pair by placing a bar over it. Since our number is 28, let us represent it as inside the division symbol.
- Step 2: We find the largest number such that when you multiply it with itself, the product is less than or equal to 28. We know that 5 × 5 = 25 as 25 < 28.
- Step 3: Let us place a decimal point and zero pairs and continue our division. Now, multiply the quotient by 2 and the product becomes the starting digit of our next divisor.
- Step 4: Choose the largest number in the unit’s place for the new divisor such that its product with a number is less than or equal to 300. We know that 0 is in the ten’s place and our product has to be 204 and the closest multiplication is 102 × 2 = 204. Therefore, use the number 2 in the unit place which gives 102 × 2 = 204.
- Step 5: Bring down the next pair of zeros and multiply the quotient 52 (ignore the decimal) by 2, which is 104, and the starting digit of the new divisor. Note that the square root of 28 is an irrational number, i.e., it is never-ending. So, stop the process after 2 or 3 more iterations by repeating steps 3 and 4, and you have the square root of 28 by the long division method.
Explore Square roots using illustrations and interactive examples
- Square Root of 22
- Square Root of 26
- Square Root of 27
- Square Root of 29
- Square Root of 30
Important Notes:
- The square root of 28 in the radical form is expressed as 2√7.
- In the exponent form, the square root of 28 is written as (28)1/2.
- The decimal representation of √28 is 5.2915.
Think Tank:
- Can you think of any quadratic equation which has its roots as √28?
- Since (√-28)2 = 28, can we say that √28 is also a square root of 28?
Source: https://t-tees.com
Category: WHICH
