(1.0) Background

The amount of water vapour in the air is critical to the weather that we experience every day. It can affect how we feel temperature. When moisture levels are high relative to the temperature, such as when fog is present (Figure 1.1), evaporation rates will be reduced, limiting the effectiveness of sweat as a cooling mechanism. Under such conditions, a given temperature may feel warmer. Atmospheric moisture is also critical to the development of storms. As clouds form, the latent heat of vaporization is released during condensation. This input of thermal energy helps drive the development of strong weather systems.

You are viewing: Which Weather Variable Can Be Determined By Using A Psychrometer

There are many ways to represent atmospheric moisture. Measures include relative humidity (RH), wet bulb temperature (Tw) and dew point (Td). Exploration of these variables also requires consideration of water vapour pressure (e). Relative humidity and dew point are commonly reported by official observation stations. They are very useful descriptions of the atmospheric moisture moisture properties at a given moment.

(2.0) Relative humidity – RH

Relative humidity is the ratio of the actual water vapour in the air over the amount of water vapour that is required for saturation given the ambient temperature. In essence, relative humidity provides an idea of how close the atmosphere is to saturation. It is as the term implies the present water vapour content of the atmosphere relative to the saturation point. An atmosphere with high relative humidity may feel moist, or muggy, while one with low relative humidity may feel dry. This is because an atmosphere with lower relative humidity is more supportive of evaporation than one with higher relative humidity, all other things such as temperature being equal. The evaporation rates of sweat and other moisture from the human body (e.g. from the mouth) respond to humidity changes, providing a sensible weather effect.

Water vapour is a gas. Dalton’s law of partial pressure informs that this gas will behave independently, adding its own pressure to the atmospheric total. The estimation of the partial pressure contribution of water vapour at a specific time is a direct way of representing the amount of moisture in the atmosphere. Given that moisture in the atmosphere is approximately 1%-ranging from ~0 to 4%-of the total gaseous constituents, water vapour partial pressures are typically low, on the order of ~1 to 40 hPa (0.03″ to 1.18″ Hg). This is far below the mean pressure of 1013.3 hPa (29.92″ Hg) at sea level, a value dominated by the key constituents of the atmosphere: diatomic nitrogen and oxygen.

Saturation is when no more water vapour can be added to the atmosphere through the process of evaporation-in basic terms. At saturation, the relative humidity is 100%, and condensation-the transfer of water vapour out of the atmosphere into liquid droplets-is likely to occur, resulting in cloud formation. This is especially true in the lower atmosphere where there may be sufficient particulate to allow condensation even at relative humidity values <100%. One way to think of saturation is that it is the maximum amount of water vapour that can exist in the atmosphere for a given temperature, save in situations of supersaturation.

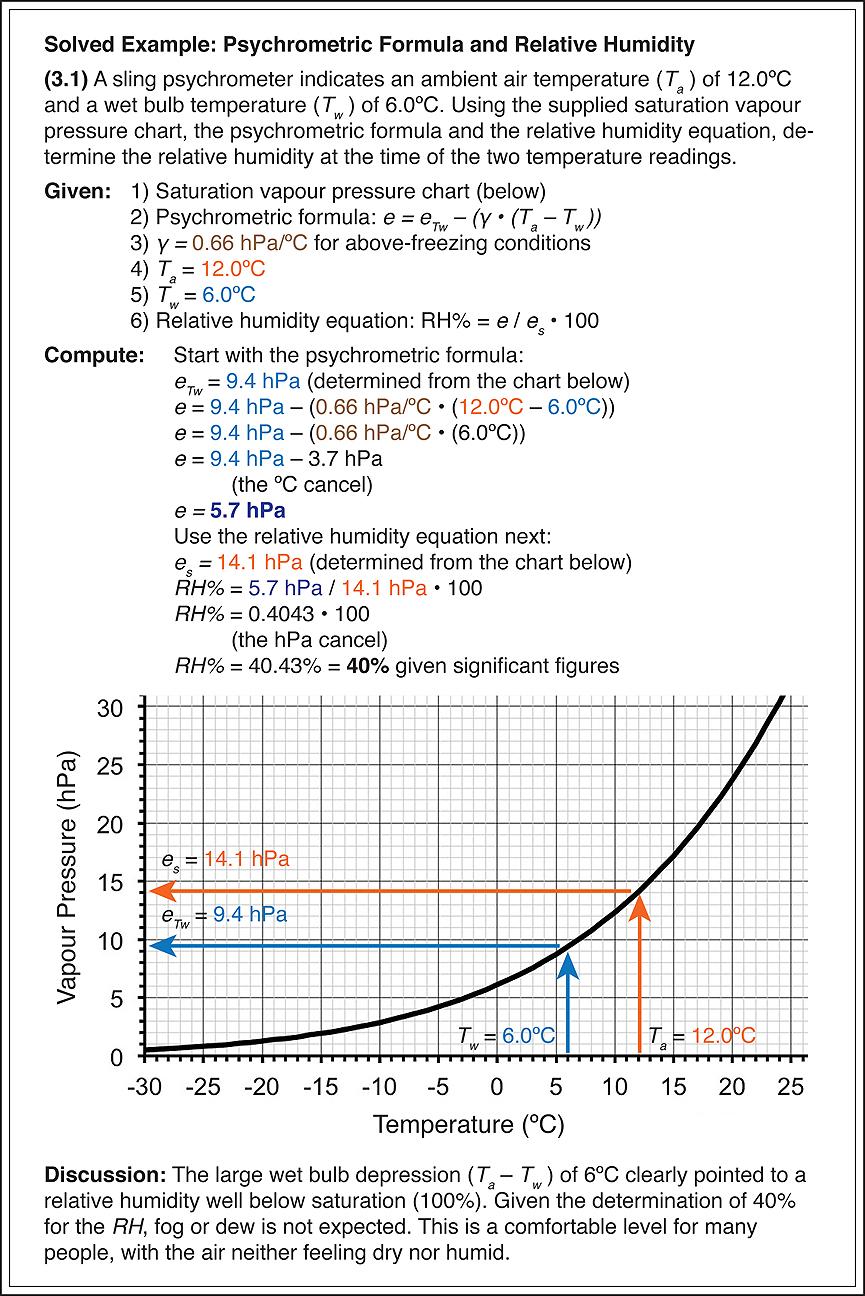
The saturation vapour pressure is the amount of atmospheric moisture-represented in terms of partial pressure-that is necessary to meet saturation conditions. For example, a given temperature on a mild afternoon, 10ºC (50ºF), has a saturation vapour pressure of 12.3 hPa (0.36″ Hg). This is the amount of water vapour required-in terms of partial pressure-for the atmosphere to be at saturation. This value is a kind of fiction. It is not the real vapour pressure, unless the atmosphere is in a state of saturation. It is a number that indicates what is required for saturation. The actual vapour pressure is often below this value. Continuing with the example, a reading of the actual vapour pressure (1.2) indicates a contribution of 10.0 hPa (0.30″ Hg). Relative humidity is determined from the two variables just described-the actual and saturation vapour pressure using (Stull 2017):

Eq 2.1) RH = (e / es ) • 100

Where RH is relative humidity in %, e is the actual vapour pressure (hPa) and es is the saturation vapour pressure (hPa)-there is much more detail on these variables in section (2.0). Using the above example, the relative humidity on a day with a 10ºC temperature, in other words having a 12.3 hPa saturation vapour pressure, and an actual vapour pressure of 10.0 hPa is: 10.0 hPa / 12.3 hPa = 0.813. Multiply by 100 to get the percentage: 81.3%. Relative humidity is always reported in percent.

(3.0) Wet bulb temperature – Tw

Wet bulb temperature is dependent on evaporation rates. Evaporation has a cooling effect due to the energy required for the phase change from liquid to vapour. High evaporation results in stronger cooling. The further the atmospheric moisture is below the saturation point, the lower the relative humidity and the stronger the cooling effect from evaporation.

The psychrometer uses two thermometers of the same design set side-by-side on a sling. A small wick, or “sock,” is put over the base of one thermometer. This sock is then moistened. Air is circulated around the two thermometers to help induce evaporation. This can be accomplished by using a handle to spin the sling, though there are fan-aspirated instruments available. After about 60 seconds, readings are taken from both thermometers. The wet-bulb depression is the difference between the ambient temperature (Ta) and the temperature from the thermometer with the sock (Tw): Ta – Tw = wet-bulb depression. For instance, if the ambient temperature is 14ºC (57ºF) and the wet bulb temperature is 10ºC (50ºF), then the wet-bulb depression is 4ºC (7ºF). Tables can be consulted to determine relative humidity from the temperature and wet bulb readings. These tables are often provided by the instrument maker, or are built into the instrument on a sliding scale.

The psychrometric formula can be used to estimate the actual water vapour pressure (e) from the wet bulb temperature (AMS Glossary 2018):

Eq 3.1) e = eTw – γ • (1 + 0.00115 • Tw) • p • (Ta – Tw)

Read more : Which Deity Am I Connected To Birth Chart

Where eTw is the wet-bulb vapour pressure in Pascals, γ is a constant of 0.00066 hPa/ºC for above freezing conditions, Ta is the ambient air temperature in ºC, Tw is the wet bulb temperature in ºC and p is pressure in Pascals. The above formula is sometimes simplified, with the assumption of sea-level pressure conditions to:

Eq 3.2) e = eTw – γ • (Ta – Tw)

Where γ is a constant of 0.66 hPa/ºC for above freezing conditions, and e is given in units of hPa (mb). Incidentally, for below-freezing conditions, γ is 0.000582 hPa/ºC for Eq 3.1 or 0.582 hPa/ºC when using Eq 3.2.

The determination of the wet-bulb vapour pressure can be done with a purpose-built chart (Figure 3.1). To do this, use the line denoting saturation vapour pressure. However, instead of using ambient temperature for your reference, use the wet bulb temperature.

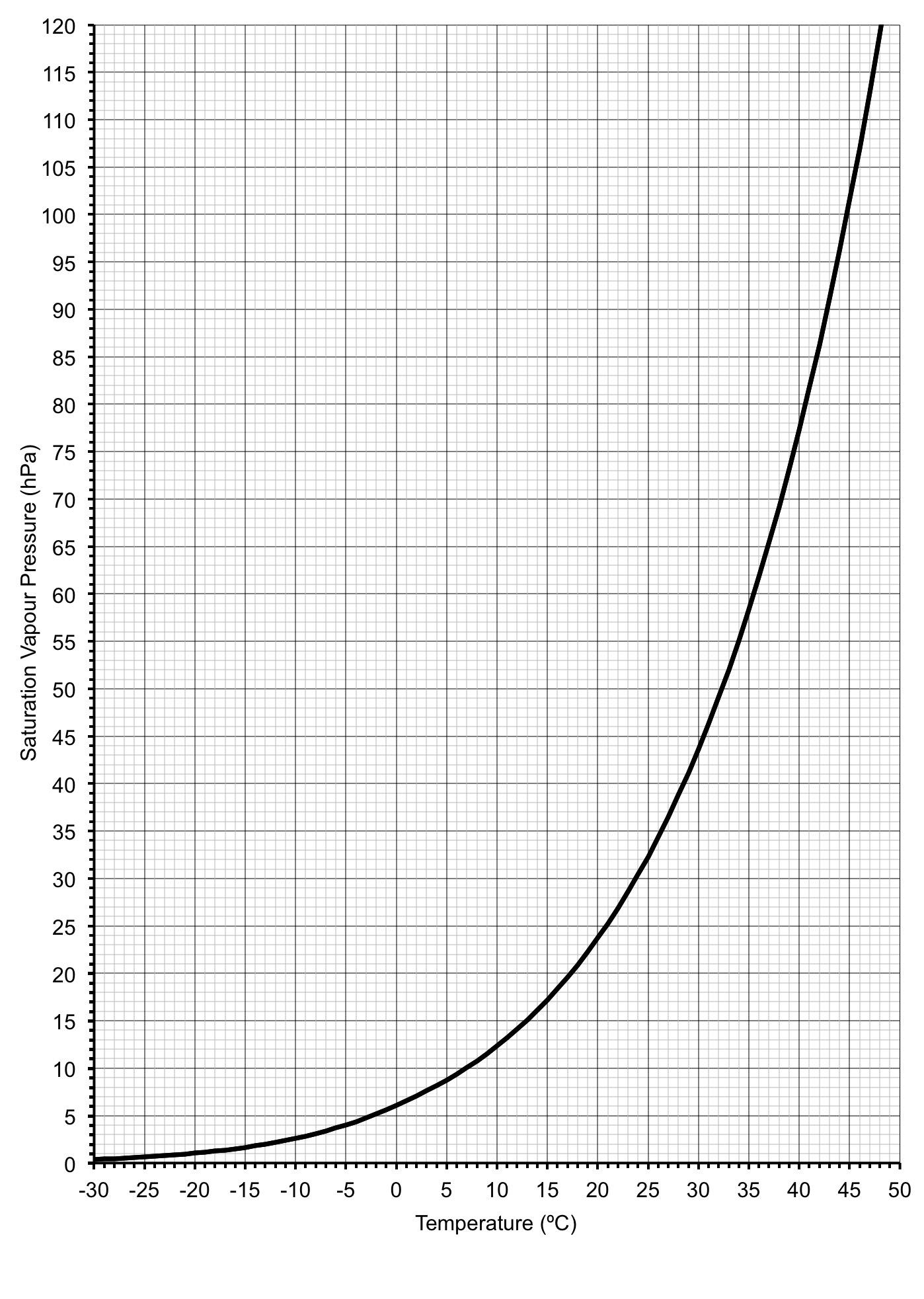
A more precise approach for determining saturation and wet bulb vapour pressures is to use the Clausius-Clapeyron equation from which the curve in Figure 1.1 has been determined (Stull 2017):

Eq 3.3) es ≈ e0 • exp ((L / Rv • (1 / T0 – 1 / T))

Where es is the approximate saturation vapour pressure, e0 is a constant equal to 0.6113 kPa, L is a latent heat parameter equal to 2.5 x 106 J / kg for liquid and 2.83 x 106 for frozen water, Rv is the water vapour gas constant equal to 461 J / (Kg • K), T0 equals the freezing point of 273.15 K and T is the ambient air temperature. The equation assumes evaporation and condensation take place from a flat water surface.

In summary, to obtain a relative humidity reading from observations of ambient air (Ta) and wet bulb temperatures (Tw), first determine the wet bulb vapour pressure (eTw) from the Tw either by using a chart (Figure 3.1), or the Clausius-Clapeyron equation (Eq 3.3). From this, the actual water vapour pressure (e) of the atmosphere can be determined by using the psychrometric formula, either Eq 3.1 or Eq 3.2. Then, by following the same procedure used to determine the wet bulb vapour pressure, estimate the saturation vapour pressure (es) by using the Ta reading. With e and es in hand, the relative humidity can be determined by using equation 2.1.

(4.0) Dew Point – Td

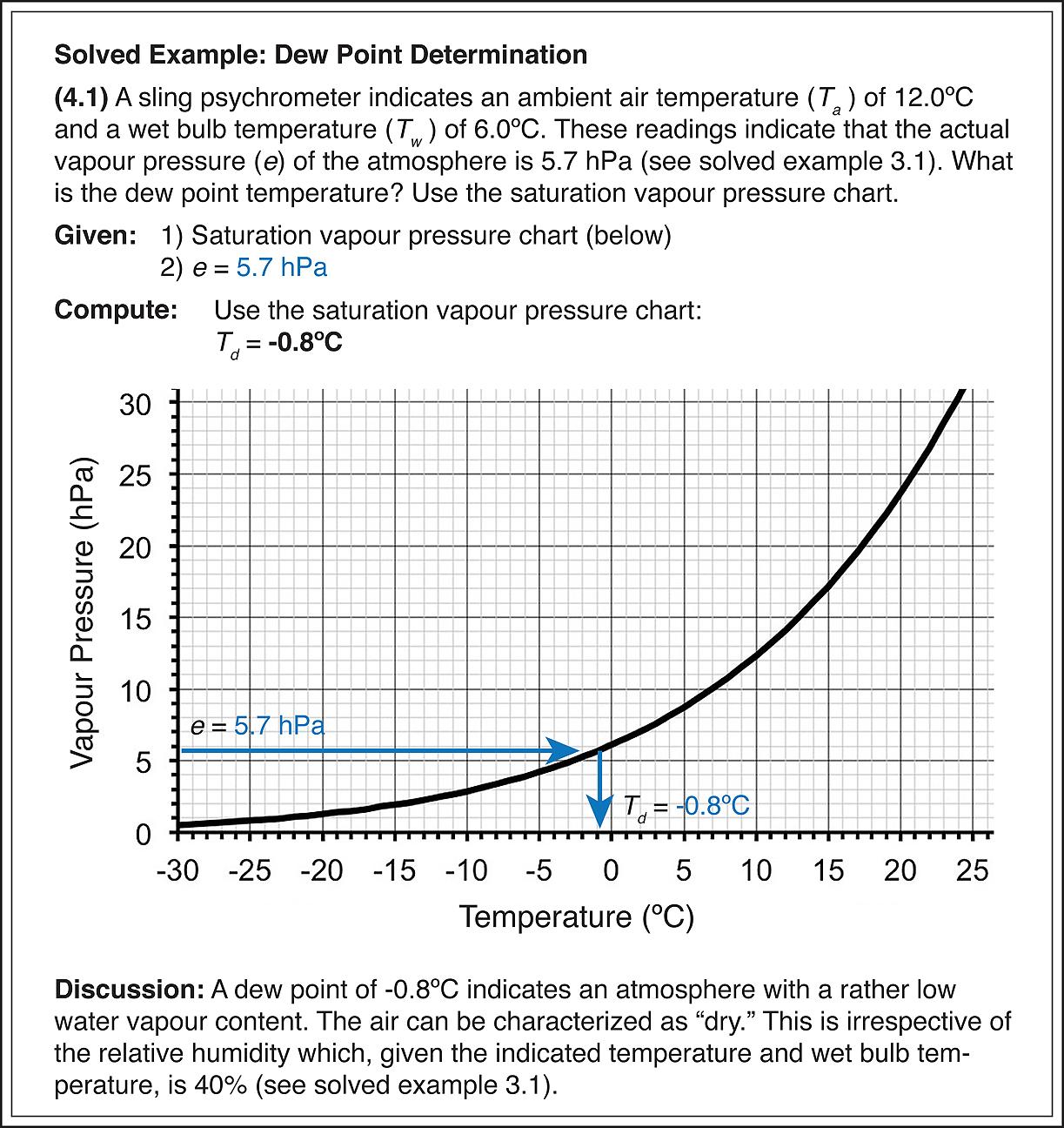
Dew point is, in essence, the saturation temperature of the air. In other words, it is the temperature that the air has to be cooled to in order to reach saturation-other conditions being held equal. Thus, the number-typically reported in ºC-is an indication of the actual amount of moisture in the atmosphere at a given time. If the temperature is 17ºC, and the dew point is 14ºC, the relative humidity is below 100% and the air is unsaturated. If this air were to cool to 14ºC without losing any water vapour, then the temperature and dew point would match, and the air would be at saturation with 100% RH. Note that even though Ta 14ºC with Td 14ºC reflects a situation with 100% relative humidity, it is erroneous to determine RH by the ratio of dew point over ambient temperature. This is because the saturation point over a range of temperatures has a nonlinear relationship.

Dew point can be determined from a psychrometer reading by using the chart in Figure 3.1. Start with the location of the actual water vapour pressure in the atmosphere-determined via the use of the psychrometric formula (3.0)-which is the y, or vertical axis on the graph. Then trace a horizontal line back to the saturation vapour pressure curve. From the intersection point on the curve, follow a line straight down to the x, or horizontal axis. Where this vertical line lands is the dew point temperature.

(5.0) Key Takeaways

(5.1) Relative humidity is the ratio of the actual water vapour to the amount of water vapour required to be at saturation for the given air temperature.

Read more : Which Is Worse Lipedema Or Lymphedema

(5.2) A psychrometer measures both the ambient air temperature and the wet bulb temperature. The measure of wet bulb temperature uses evaporational cooling that is caused by the energy uptake requirement to make the phase change from from liquid to gas. The lower the relative humidity, the higher the evaporation rate and the lower the wet bulb temperature.

(5.3) The difference between the ambient and wet bulb temperature is called the wet bulb depression.

(5.4) The actual water vapour content of the atmosphere, measured in terms of partial pressure (hPa), can be determined from the ambient air temperature and wet bulb readings by using the psychrometric formula.

(5.5) The Clausius-Clapeyron formula can be used to estimate the saturation vapour pressure for a given temperature. This is used to create the saturation vapour pressure chart that is useful for determining actual water vapour pressure from psychrometer readings.

(5.6) The dew point temperature is the temperature at which the atmosphere has to be cooled to for saturation to be reached, provided the there is no transfer of moisture from the air during the cooling process. Dew point can be determined with the saturation vapour pressure chart if the water vapour pressure in the atmosphere is known.

(6.0) Exploration

(6.1) If the temperature is -15ºC and the dew point is -15ºC, the relative humidity is 100%. What is the wet bulb reading? Is the atmosphere “dry” or “moist” under this situation? Explain your answer.

(6.2) If the temperature is 50ºC and dew point 20ºC, the relative humidity is 26%. Is the atmosphere “dry” or “moist” under this situation? Explain your answer.

(6.3) In terms of hPa, how much more water vapour is in the atmosphere in (6.2) when compared to (6.1)? Under which situation is atmosphere moist? Explain your answer.

(6.4) What problems might arise when a sling psychrometer is used under below-freezing conditions?

References

AMS Glossary, 2018: American Meteorology Society Glossary of Meteorology, accessed at here on 19 October 2018.

Stull, R., 2017: “Practical Meteorology: An Algebra-based Survey of Atmospheric Science” -version 1.02b. University of British Columbia. 940 pages.

ATSC 201 (4): Relative Humidity, Wet Bulb Temperature and the Psychrometric Equation

Began: 17 October 2018 Modified: 21 October 2018

Source: https://t-tees.com
Category: WHICH